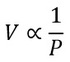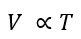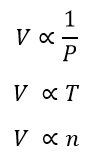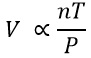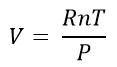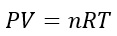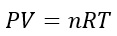The Ideal Gas Equation

If you pump too much air into a party balloon, the pressure of the air inside will burst it with a loud bang. Put one into the fridge and it will shrink a bit. You have carried out two simple experiments into the behaviour of gases. Over three centuries ago, scientists were carrying out careful measurements to see what gases do if their pressure or temperature is changed.

Boyle’s law Robert Boyle, the author of The Sceptical Chemist, published the results of his experiments on gases in 1661. Boyle discovered that as long as the temperature of a gas stayed the same, the higher the pressure the smaller its volume. Boyle’s law states that:

The volume of a fixed mass of gas (at a constant temperature) is inversely proportional to its pressure.

This can be shown using the equation:

​This marshmallow is under normal atmospheric pressure, so the air bubbles inside it are the expected size.
This marshmellow is under reduced atmospheric pressure, so the air bubbles inside it have expanded and hence the marshmallow has increased in size.

The Magdeburg hemispheres

Robert Boyle’s experiments were greatly helped by Otto von Guericke’s invention of the vacuum pump in 1650. Boyle could easily change the pressure exerted on a sample of gas using a vacuum pump. But the inventor himself did something quite amazing using such a pump. Von Guericke carried out a famous experiment in Magdeburg in 1654 in front of the Holy Roman Emperor (Ferdinand III) and a crowd of very impressed people. He pressed two copper hemispheres together and pumped the air out. Two teams of eight horses could not pull them apart. Air pressure pushed the hemispheres tightly together until air was let back in.

Charles’s law

Jacques Charles investigated the relationship between the temperature of a gas and its volume. The French scientist released his results in 1787. Charles’s law states that: The volume of a fixed mass of gas (at a constant pressure) is proportional to its absolute temperature. This can be shown using the equation:

Where V is the volume and T is the absolute temperature (in Kelvin)

Putting it all together

By 1811 there were three gas laws:

The bottom equation is known as Avogadro’s principle and here n is the number of moles and volume is proportional to this.

All of these equation can be combined:

We do not normally express equations with a proportionality sign, so to remove this we need to add a constant and the expression becomes:

Where R is the gas constant (8.314 JK-1mol-1). This same equation is usually expressed in its more familiar form and is known as the Ideal Gas Equation:

  • P is the pressure measured in pascals, (Pa)
  • V is the volume measured in cubic metres (m3)
  • n is the amount of gas measured in moles (mol)
  • R is the gas constant. It is 8.31 JK-1mol-1 (joules per kelvin per mole)
  • T is the absolute temperature measured in kelvin (K)

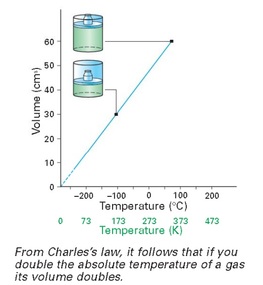
Absolute temperature

If you study the graph of volume against temperature, you will see that there is a temperature at which the gas would have zero volume. This is called absolute zero. It is a very chilly −273.15 °C. The absolute temperature scale starts at this temperature. Absolute temperature is measured in kelvin, K. The great thing about the absolute temperature scale is that there are no negative temperatures. This makes any calculations much easier to do. A change of 1 K is the same as a change of 1 °C. You would say “one kelvin” and not “one degree Kelvin”.

Kinetic Theory assumptions about ideal gases

There is no such thing as an ideal gas of course, but many gases behave approximately as if they were ideal at ordinary working temperatures and pressures. The assumptions are:

  • Gases are made up of molecules which are in constant random motion in straight lines.
  • The molecules behave as rigid spheres.
  • Pressure is due to collisions between the molecules and the walls of the container.
  • All collisions, both between the molecules themselves, and between the molecules and the walls of the container, are perfectly elastic. (That means that there is no loss of kinetic energy during the collision.)
  • The temperature of the gas is proportional to the average kinetic energy of the molecules.

And then two absolutely key assumptions, because these are the two most important ways in which real gases differ from ideal gases:

  • There are no (or entirely negligible) intermolecular forces between the gas molecules.
  • The volume occupied by the molecules themselves is entirely negligible relative to the volume of the container.

The Ideal Gas Equation

As mentioned already, the ideal gas equation is:

Exploring the various terms

Pressure: In this equation it is measured in pascals (Pa) but is sometimes expressed as newtons per square metre, Nm-2. These mean exactly the same thing. Be careful if you are given pressures in kPa (kilopascals).

For example, 150 kPa is 150,000 Pa. You must make that conversion before you use the ideal gas equation. Should you want to convert from other pressure measurements:

1 atmosphere = 101,325 Pa                   1 bar = 100 kPa = 100,000 Pa

Volume: This is the most likely place for you to go wrong when you use this equation. That's because the SI unit of volume is the cubic metre, m3 - not cm3 or dm3.

1 m3 = 1000 dm3 = 1,000,000 cm3

So if you are inserting values of volume into the equation, you first have to convert them into cubic metres. You would have to divide a volume in dm3 by 1000, or in cm3 by 1 million. Similarly, if you are working out a volume using the equation, remember to covert the answer in cubic metres into dm3 or cm3 if you need to - this time by multiplying by a 1000 or 1 million. If you get this wrong, you are going to end up with a silly answer, out by a factor of a thousand or a million. So it is usually fairly obvious if you have done something wrong, and you can check back again.

Number of moles (n): To use the equation you will quite often first be expected to calculate the number of moles, and you do this by taking the mass in grams and dividing it by the Mr.

The gas constant (R): A value for R will be given to you if you need it, or you can look it up in a data source. The SI value for R is 8.31441 JK-1mol-1.

The temperature (T): The temperature has to be in Kelvin. Don't forget to add 273 if you are given a temperature in degrees Celsius.